Periodic Interest Rate: Meaning, Examples, Advantages, Excel Calculation, and More

Periodic Interest Rate: Meaning

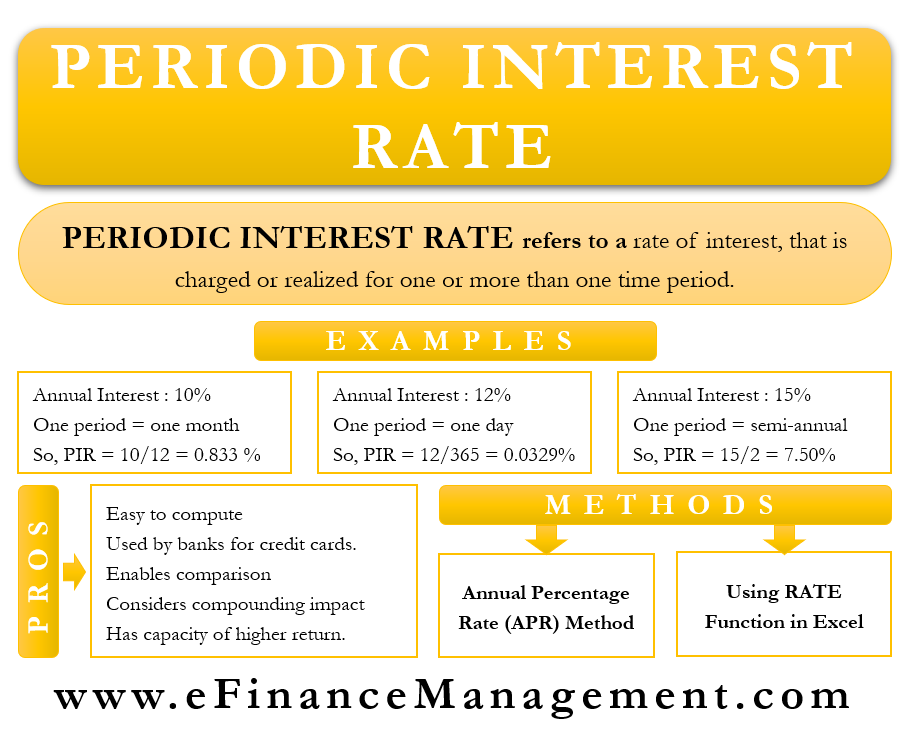
A Periodic Interest Rate is a rate of interest that is charged or realized for one or more than one time period. Depending on the situation, the time period can be a week, month, quarter, or any particular time frame. All the time periods are of equal time length, i.e., if Period 1 is for 60 days, then Period 2 will also be for 60 days.

Periodic Interest Rate demonstrates the compounding nature of the loan or an investment. It considers the interest rate for every time period it is charged or realized, as defined in the agreement. The more compounding periods, the higher will be the effective rate of return or interest at the end of the financial year. Let’s say Investment A gives a 10% annual interest rate, compounding semi-annually, and Investment B gives a 10% annual interest rate, compounding monthly. In such a scenario, Investment B gives a higher effective interest rate than Investment A.

Periodic Interest Rate: Examples

Mostly, the lender or issuer quotes the interest rate on an annual basis. To come up with a periodic interest rate, the annual interest rate is divided by a number of periods in a year.

Example 1

Let’s say the annual interest rate is 10%, and one period is of a month. In such a situation, the total number for periods in a year would be 12, and the calculation will be as follows:-

10/12 = 0.83%

In the above example, the monthly Periodic Interest Rate will be 0.83%.

Example 2

Let’s say the annual interest rate is 12%, and one period is for a day. In such a situation, the total number of periods in a year would be 365, and the calculation will be as follows:-

12/365 = 0.0329%

In the above example, the daily Periodic Interest Rate will be 0.0329%.

Example 3

Let’s say the annual interest rate is 15%, and the interest is compounded semi-annually, with a total of two periods in a year.

15/2 = 7.5%

In the above example, the semi-annual Periodic Interest Rate will be 7.5%.

Advantages of Periodic Interest Rate

• This interest rate helps in the easy computation of interest rates realized or charged for a particular compounding period. Credit Cards use this concept in a very effective manner.
• This interest rate is also very useful when the lending or investments are made for a duration of less than a year, which helps in easy computation and realization of returns or repayments in such a situation.
• This interest rate helps the investor or the borrower to compare various financial instruments to get better results.
• It considers the compounding effect on the loan or investment and also comes up with an effective annual interest rate.
• If the interests or profits accrued are not realized and are reinvested, then it has the capacity to give higher long-term returns on the investments. These advantages are non-exhaustive in nature.

Periodic Interest Rate Vs Annual Percentage Rate (APR)

There are many differences between these two terms; they are as follows:-

Periodic Interest Rate	Annual Percentage Rate (APR)
This rate of interest is quoted periodically.	APR, as the name suggests, is quoted annually.
This interest rate takes the compounding effect into consideration.	APR does not take the compounding effect into consideration.
Mostly, Periodic Interest Rate is more accurate in comparison to APR. It gives the actual cost of borrowing or actual returns on investments.	APR is comparatively less accurate as there are high chances of understating the cost of the loan or overstating the returns because of its long-term schedule.
This is mostly for a shorter period of time.	This is mostly for a longer period of time.

These differences are non-exhaustive in nature.

Calculation of Periodic Interest Rate in Excel

There are quite a few methods for calculating Periodic Interest rates in Excel, but the following are two major methods:-

APR Method

This is the basic method for calculating this interest rate in excel. This method is useful when the total number of periods in a year and the Annual Percentage Rate (APR) are given. This interest rate is calculated after dividing the APR by the total number of periods in a year. Let’s understand this with an example.

	A	B	C	D
1	Annual Percentage Rate (APR) (%)	Interest Rate Compounded	Number of Periods in a Year	Periodic Interest Rate (%)
2	10	Weekly	52	=APR/52 (Where the cell A2 will be divided by cell C2)
3	11	Monthly	12	=APR/12 (Here the, cell A3 will be divided by cell C3)
4	12	Semi-Annually	2	=APR/2 (Where the cell A4 is divided by the cell C4)

As shown in the above example, Periodic Interest Rate is calculated by dividing APR by the total number of periods in a year in an Excel Sheet.

Using RATE Function

The second major method useful for the computation in Excel is with the help of the RATE function of Excel. Using of RATE function can be taken place in two major ways, as follows:-

Periodic Payment is given

In this scenario, the Loan amount or investment amount (PV) is available, the total number of periods in a year is available (nper), and Periodic payments/repayments (pmt) are also available. With the help of the RATE function, the computation takes place. Let’s understand this with an Excel Sheet example.

	A	B
1	Loan/Investments ($) (PV)	20000
2	Total number of Periods in a Year (Compounding Monthly) (nper)	12
3	Periodic Payments/Repayments ($) (pmt)	-2000
4	Rate (Periodic Interest Rate) (%)	3% (Where =RATE(nper,pmt,pv) = 3% Application of cell B2,B3 and B1 takes place under RATE function

In the above RATE function, it is compulsory to record Periodic Payments in Negative.

Periodic Payment is not given

There are times when the Periodic Payments/Repayments (pmt) is not available. In such a situation, Future Value (FV) is used in the RATE function for computation.

	A	B
1	Loan/Investments ($) (pv)	-20000
2	Total number of Periods in a Year( Compounding Monthly)(nper)	12
3	Future Value ($) (fv)	25000
4	Rate (Periodic Interest Rate) (%)	2% (Where=RATE(nper,,pv,fv) = 2%) Application of cell B2,B1 and B4 takes place under the RATE function

While using the above RATE function, the loan amount or investment amount (pv) should be negative, and the RATE function should be =RATE(nper,pv,fv).

Conclusion

Periodic Interest Rate has become a very popular term in the financial services industry. It is extensively useful in the lending industry for accurate calculations and showcasing the compounding effect. The functioning of Credit Cards thrives on this concept only. This Interest Rate helps the investors and the borrowers compute the actual cost of borrowing and actual return on investment, respectively. When the lending or investments is for a shorter duration or a compounding effect is seen, this interest rate is useful. Irrespective of minimal criticisms, it is one of the best useful tools.

Continue reading to learn more about various other Types of Interest Rates.