Annuity formula as a standalone term could be vague or ambiguous. It can be either ‘present value annuity formula‘ or ‘future value annuity formula.’ Before we learn how to use the annuity formula to calculate annuities, we need to be conversant with these terms.
What is Annuity?
It is a series of periodical payments or receipts of a fixed amount for a specified period. For example, a contract specifying $1500 of rent payable monthly for 5 years. This is an annuity payment. Here, the fixed amount is $1500; periodical payments are monthly payments for up to 5 years, i.e., 60 payments (12*5), and the specified period is 5 years.
Common examples of annuity payments are rent paid for rental properties or installments paid against the borrowed loan. On the other hand, annuity receipts arise, in the case of a certificate of deposit, interest on a bond where you receive a series of payments.
There are various ways of computing the worth of such payments. Hence, you must understand the concept of the present value or future value of an annuity.
Also Read: Ordinary Annuity vs Annuity Due
Before that, you must know about an ordinary annuity and annuity due and their difference.
Future Value of Annuity
The future value of annuity measures the value of the series of the recurring payments at a given point of time in the future at a specified interest rate.
Suppose Mr. John owns a bungalow and he rented it to Mr. George for 3 years. George finds paying the rent every month very inconvenient. He asks Mr. John to tell him a lump sum amount to be paid at the end of 3 years to avoid monthly payments. Mr. John needs to find out a lump sum whose value is equivalent to receiving rent for 3 years. This value is the future value of the annuity.
Present Value of Annuity
The present value of the annuity calculation helps to know the present worth of recurring fixed annuity payments in the future.
Suppose Mr. John owns a bungalow and he rented it to Mr. George for 3 years. George finds paying the rent every month very inconvenient. He asks Mr. John to tell him a lump sum amount to be paid now, i.e., beginning of 3 years, to avoid monthly payments. Mr. John needs to find out a lump sum whose value is equivalent to receiving rent for 3 years. This value is the present value of the annuity. We will learn how to calculate this in this article.
Types of Annuity
Annuities are of two types:
Ordinary Annuity
An ordinary annuity is an annuity receipt or payments that occur at the end of each period of the specified time. Example interest payments of the bond, home mortgage payments, etc. Under this type of annuity, if there are monthly payments, it is assumed that they are paid at the end of each month. i.e., 31st Jan, 28th Feb, 31st Mar, and so on.
Also Read: Present Value of Annuity
Annuity Due
An annuity due is annuity receipts or payments that occur at the beginning of each period of the specified time. Example rents are generally payable to the landlord at the beginning of every month. In case of an annuity due, if there are monthly payments, we assume the payment to be done on 1st Jan, 1st Feb, 1st Mar, and so on.
Annuity Formula and its Calculation
Now, we will explain 4 formulas in total
- Future Value of Ordinary Annuity
- Future Value of Annuity Due
- Present Value of Ordinary Annuity
- Present Value of Annuity Due
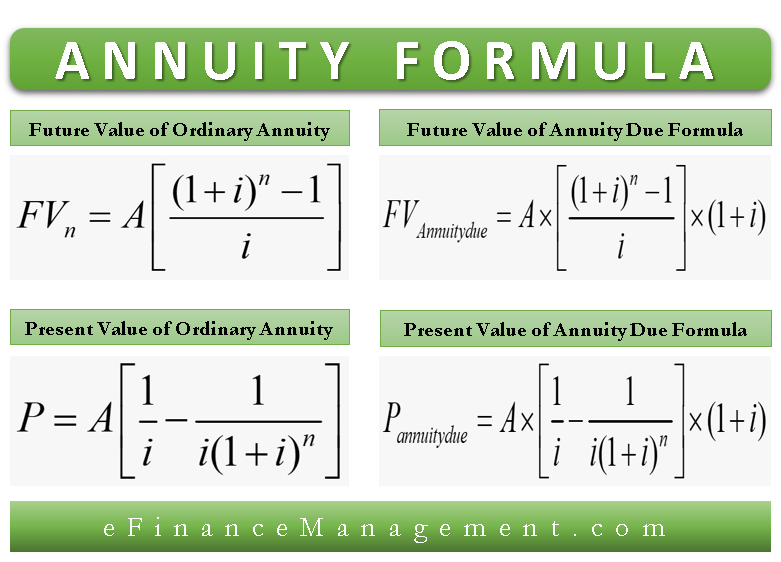
Future Value of the Ordinary Annuity Formula
Formula
We can use the following formula to calculate the future value of an ordinary annuity, abbreviated as FVn.
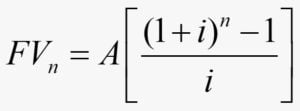
here,
A = annuity cash flow, i = interest rate, n = number of payments.
Calculation with Example
ABC ltd. deposits a fixed amount of Rs. 5000 at the end of each year for three years at an interest rate of 6%. How much worth will be these annuities would accumulate at the end of the third year?
Now, there are 3 ways, we can calculate it
Calculation using Formula
5000[((1+6%)3-1)/6%] = 5000[((1+0.06)3-1)/0.06]=5000*((1.063-1)/0.06)= 5000*((1.191-1)/0.06)=5000*((0.191)/0.06)=5000*3.1836=15918
Manually for Small Periods
If the period is, say, 3-5 years, you may manually calculate it.
What does it imply?
It implies that Rs. 5000 deposited in the first year will yield interest for 2 years, Rs 5000 in the second year for 1 year, and in the third year, Rs.5000 deposited will not yield any interest. The compounded value of each payment is calculated and added to arrive at the future value of an annuity.
FV3= 5000[{(1+6%)3-1/6%}]= 15,918.
Using Compound Table
We can also calculate using table values of compound value factor of an annuity of Re. 1, also known as (CVFAn.i) table
The formula is:
FVn = Annuity Cash flow × CVFAn,i
here,
CVFAn,i = Compound value factor of an annuity of Re 1 for n number of years at i rate of interest.
CVFA3,6 = 3.184
So, FV3 = 5000 x 3.184 = 15,920.
Note: The answer varies slightly with different formulas due to rounding off the digits to the nearest decimal.
Read about the Present Value Table in detail.
Present Value of an Ordinary Annuity Formula
The time value of money concept is used for calculation that says any sum is now worth more than it will be in the future as you can invest it somewhere else. So, the first payments are worth than the second, and so on.
Formula
We can use the following formula to calculate the future value of ordinary annuity abbreviated as P.
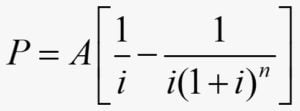
here,
P = Present value of annuity, A = Annuity cash flow, i = rate of interest, n= number of payments.
Calculation with Examples
Suppose a person receives an annuity of Rs. 5000 for 3 years. Suppose the rate of interest is 10 percent, the present value of Rs. 5000 annuity is:
Calculation using Formula
P= 5000[{1/10%-1/10%(1+10%)3}] = 12,450
So, the present value of the ordinary annuity is Rs. 12,450
Future Value of Annuity Due Formula
Recalling what distinguishes an annuity due from an ordinary annuity is the time of payments of the annuity. Since payments of the annuity due are made at the start of each period. So, there is a slight change in the formula for computing the future value.
Formula
We can use the following formula to calculate the future value of an annuity due, abbreviated as FVannuity due.
here,
FVannuity due = Future value of annuity due, A = Annuity cash flow, i = rate of interest, n= number of payments.
Calculation with Example
Understanding the calculation of FV, the annuity due using the same example of the future value of an ordinary annuity:
Calculation using Formula
FV3(annuity due)=5000[{(1+6%)3-1/6%} x (1+6 %)]=16,873.08
Note: The future value of an annuity due for Rs. 5000 at 6 % for 3 years is higher than the FV of an ordinary annuity with the same amount, time, and rate of interest. This is due to the earlier payments made at the starting of the year, which provides an extra time period to earn more interest. For example, the payment of Rs. 5000 is invested on April 1 rather than April 31; it has an additional month to yield interest
Present Value of an Annuity Due Formula
Early payments make a difference in amounts, as we saw in the case of the future value of the annuity due. Hence, the formula for the present value of an annuity due also changes because of the beginning payments of the annuity.
Formula
We can calculate the present value of annuity due payments using the following formula:
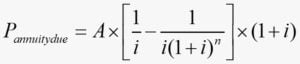
here,
Pannuity due = Present value of the annuity due, A = Annuity cash flow, i = rate of interest, n= number of payments.
Calculation with Examples:
Calculating the PV of the annuity due using the same example of the present value of the ordinary annuity:
Pannuity due= 5000 x [{1/10%-1/10%(1+10%)3}] x 1.10 =13,695
Thus, the PV of the annuity due is 13,695.
Final Words
You can also calculate the future value or present value of annuities using excel formulas under the head financial from the formula tab and even can use financial calculators. The financial calculators are available online and make the calculating part easy, provided you enter the correct figures.
Continue reading – Capital Recovery Factor

