Spot Interest Rate

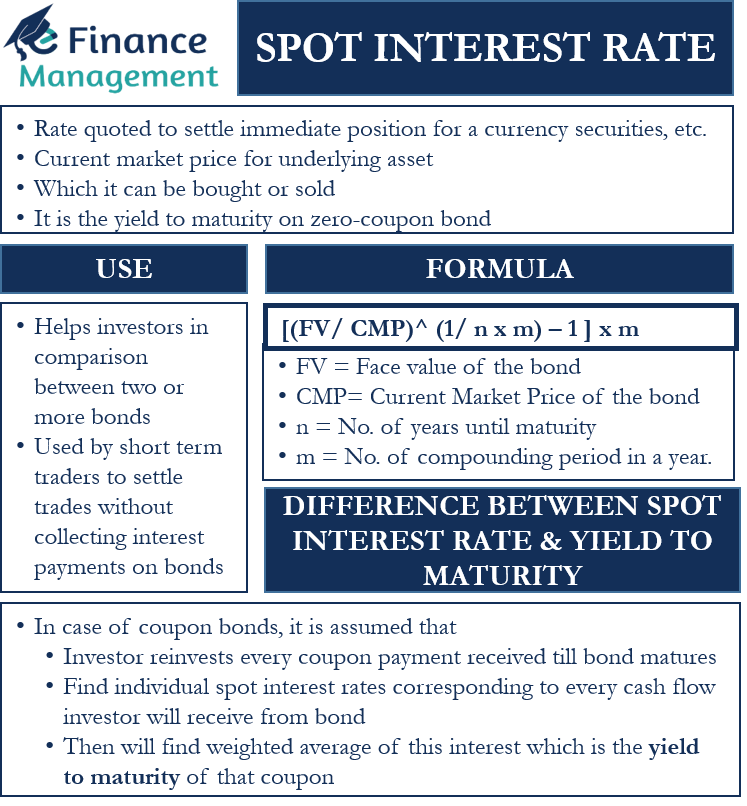
What do we Mean by the Spot Interest Rate?

Spot interest rate is the yield to maturity on any zero-coupon bond with an “x” number of years until maturity. It is the current or present market price for any underlying asset at which it can be bought or sold. In common parlance, we mention the spot interest rate as a rate for the maturity of that “x” number of years.

A zero-coupon bond is a bond where the investor does not get the interest during the tenure of the bond. It is often issued at a deep discount to face value to lure investors into investing in it. The holders get the face value of the bond at maturity. To measure the rate of return that an investor will require until the maturity period, we calculate the yield for the tenure. In a way, It is actually the present value of the face value of the bond. The spot interest rate is the YTM or yield-to-maturity of such zero-coupon bonds. Therefore, in simple words, it is the rate of return that an investor can earn on bonds when he trades them at a particular point of time without collecting interest on them.

How do we Use the Spot Interest Rate?

Spot interest rate is of use to investors to help them compare between two or more bond offerings. Short-term traders and market makers use it to settle their trades without collecting the interest payments on the bonds. From the point of view of the buyers of bonds, it is important when they are purchasing the bond from some other seller in the secondary market. They can compare this interest rate of the various bond options available for investment and choose among them. The sellers of such instruments can correctly price their offerings and get the best price for the same.

A holder of a zero-coupon bond technically does not earn interest from the bond. However, he does earn implicit interest on the same. This is because the price of the bond automatically moves northwards towards its face value as it gets closer to maturity. Thus, the spot interest rate denotes the change in the price of the bond over time, even though the bond is zero-coupon and does not pay out any interest over its lifetime.

Calculation and Example

The formula for calculation is as follows:

[(FV/ CMP)^ (1/ n x m) – 1 ] x m

Here, FV = Face value of the bond, CMP= Current Market Price of the bond, n= number of years until maturity, and m = number of compounding periods in a year.
Let us assume that we have a bond with a face value of $100 and have fifteen years until maturity. The CMV or current market value of the bond is $40. We can use the above formula to calculate the spot interest rate as follows:
($100/$40) ^1/15 -1
=1.063-1
=6.3%

In the above example, let us now assume that the bond is with bi-annual compounding. We will calculate the spot interest rate in this case as follows:
[($100/$40) ^(1/15×2) -1] x 2
= (1.030 -1) x 2
=6.14%.

Therefore, we see that the spot interest rate with bi-annual compounding is slightly lower than that with interest being compounded annually. This indicates that the spot interest rate also varies with the number of compounding periods in a year.

What is the Difference between Spot Interest Rate and Yield to Maturity?

As discussed above, the spot interest rate and yield to maturity are the same in the case of zero-coupon bonds. However, for interest-paying bonds, both these are different and not the same. In the case of such bonds, we assume that the investor reinvests every coupon payment he receives at an average interest rate until the bond matures. We first find out the individual spot interest rates that correspond to every cash flow that an investor will receive from the bond. We then find out the weighted average of these interest rates. This weighted average is the yield to maturity of the bonds that pay coupons or regular interest.

Let us understand this with the help of an example. Let us assume that a bond with a face value of $100 has a spot interest rate of 8% for the first year and 10% for the second year, respectively. The spot interest rates for the second year and further years are usually higher, keeping in mind that the inflation will increase over the years. Now suppose that the bond pays a 5% coupon. To find the present value of this bond, let us treat it as two separate zero-coupon bonds- one maturing in one year and the other maturing in the next year.

Present Value= $5/ 1+.08 + $105/ (1+.10)^2
=$91.41

To calculate the yield to maturity of the bond, let us find the value of x in the following equation-
$91.41= $5/ (1 +x) + $105/ (1 + x)^2
Here, we get the value of x as 9.95%.

This is the yield to maturity of this bond.

Also, read – Spot and Forward Interest Rate.