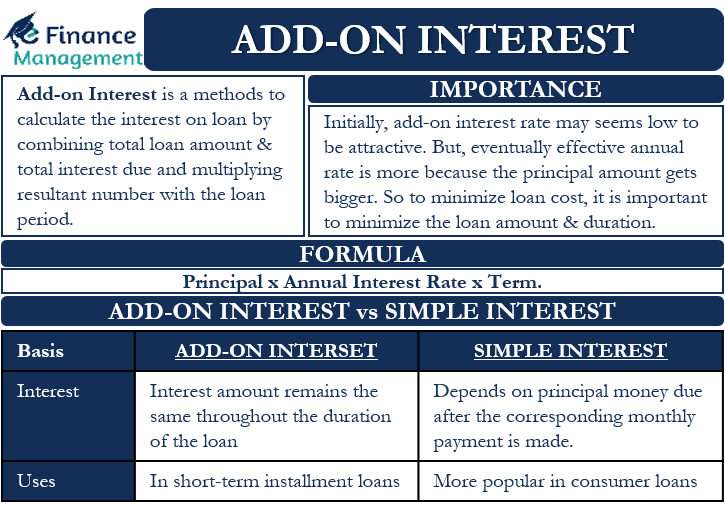
As we know, there are several methods to calculate interest. And Add-on Interest is one of these methods to calculate the interest on a loan. In this method, we calculate the interest on a loan by combining the total loan amount and the total interest due. We then multiply that resultant number with the loan period. Then, we divide this total payable amount over the loan period by the number of monthly payments to get the EMI amount for the loan.
Basically, this method combines the principal amount and the total interest for the loan period to get one single amount. This method ensures that the lender gets all the interest even if the borrower pays back the loan earlier because the total interest for the loan period has already been added to the principal amount while calculating the EMIs.
The formula to calculate the monthly payment using the add-on interest method is:
(Principal + Interest for the total period) / Total Number of Payments.
Here, we calculate the interest using the following formula= Principal x Annual Interest Rate x Term (number of years, etc.).
For example, assume Mr. A takes a two-year loan of $1,000 at an interest rate of 9%. In the case of the add-on interest method, the total simple interest for two years will be $180. Thus, the total due amount will be $1,180. This means Mr. A will have to pay $590 per year.
Importance of Add-on Interest
Let us understand the importance of add-on interest rate and calculation thereof with an example.
Suppose Mr. A takes a loan of $500,000 to buy a house. And the total loan period is five years and is available at an add-on interest of 10% p.a.
So, the total interest for five years will be 50% (10%*5), or $250,000 for five years. Therefore, the total due amount will be $750,000. Moreover, as the loan is for 5 years (60 months), the monthly payment that Mr. A will have to make is $12,500.
Many may not worry about paying a 10% add-on interest rate. But, if we consider the effective rate that we will be paying, then we may be surprised. The effective rate is basically the real rate that we are ultimately paying on the outstanding loan amount after deducting the regular principal repayment. In the above case, the effective interest rate comes out to be 17.27%.
On the face of it 10% add-on interest rate looks quite lucrative. However, Mr. A is actually paying an interest rate of over 17% by this method. This is because the 10% interest rate is getting applied to the original principal amount even when Mr. A is regularly making payments of the principal amount (via EMI).
In contrast, when the bank pays interest on savings, they usually quote the effective interest rate.
Thus, it is important that we are aware of what the bank or any other financial institution is effectively charging us. Initially, we may find a low add-on interest rate to be attractive. But, we will eventually end up paying more because the principal amount gets bigger. Thus, to minimize our loan cost, it is important to minimize the loan amount and the duration of the loan.
Add-on Interest vs Simple Interest
The add-on interest method of calculating interest is more expensive for the consumers as they end up paying more. The above example has made it amply clear as the amount of outstanding interest remains the same throughout the duration of the loan. Therefore, this method of interest calculation is not popular in consumer loans. But, financial institutions may use this method in short-term installment loans, auto loans as well as in loans to subprime borrowers.
Most loans use the simple interest method. In the simple interest method, the interest calculation is on the basis of principal money due after the corresponding monthly payment is made, i.e., on the net outstanding balance. So, if a borrower pays down a simple interest loan early, it results in significant savings. This is because all the future monthly payments (and interest on it) translate into savings.
Though the EMI will remain the same in all cases, however, in simple interest loans, the amount towards principal increases and interest drops from the same EMI amount as the loan period progresses. On the other hand, the interest paid remains the same in the add-on interest method. So the split between principal and interest remains the same throughout the loan duration in the case of Add-on interest loans.
The below example will help to bring out the difference between the two clearly.
Mr. A takes a loan of $25,000 carrying an 8% interest rate for four years.
- So, if the add-on interest rate is applicable, then the total interest to be paid by Mr. A over the 4 year loan period would be $8,000 ($25,000 x 8% x 4).
- However, in the case of simple interest, the total interest will be only $3,586. This means Mr. A will be paying $4,414 more by going for an add-on interest rate. Obviously, the effective interest rates turn out to be more than double of the add-on interest rate of 8%.
Final Words
It is crucial for borrowers to understand the concept of the add-on interest method. Knowing this concept would help them to be aware of the interest method the financial institution is going to apply. If we are not sure about the method we are being charged, it is always better that we clarify this point and well understand the effective interest rate before signing any loan documents.
Frequently Asked Questions (FAQs)
The formula to calculate the monthly payment using the add-on interest method is:
(Principal + Interest for the Total Period) / Total Number of Payments.
Where interest for the total period = Principal x Annual Interest Rate x Term (number of years, etc.).
It calculates interest on the original amount borrowed and not on the amount reduced by any repayments.

