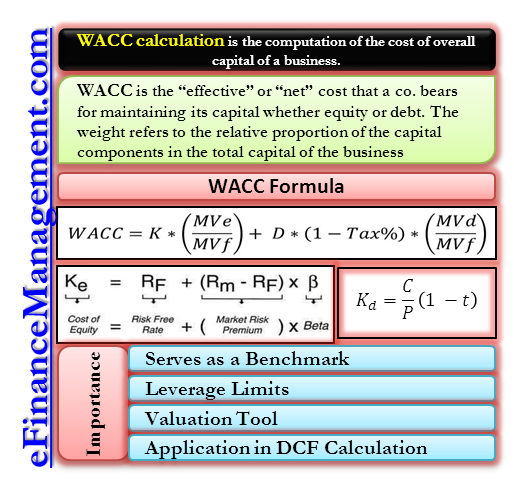
How to calculate WACC is calculation is the computation of the cost of the overall capital of a business. The capital structure of a business comprises components of debt and equity, which have been procured at different costs. The calculation of WACC gives an aggregated and all-inclusive cost that is computed after taking into account the varying cost structure of all the capital components.
WACC or Weighted Average Cost of Capital is the “effective” or “net” cost that a business bears for maintaining its capital, whether equity or debt. The weight refers to the relative proportion of the capital components in the business’s total capital. The cost of total funds of a business cannot be known by studying the capital components in isolation. The true picture emerges when they are studied collectively under a single lens. That is exactly the purpose of a WACC calculation. After all, the value of any enterprise is the combined effect of both equity and debt on its balance sheet. WACC calculation aggregates the various sources of funds. This provides a singular yet all-encompassing number to judge the cost-effectiveness. Lets see how to calculate WACC using formula in detail.
WACC Formula
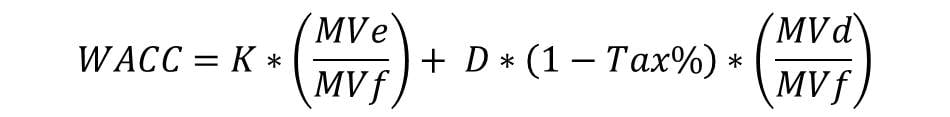
Or the extended formula looks like this:
WACC =Cost of Equity * % of Equity+ Cost of Debt(1-t) * % of Debt+ Cost of Preferred Stock * % of Preferred Stock
Breaking down the Formula
To appreciate the WACC calculation in its entirety, it helps to understand the derivation and rationale behind its components.
Cost of Equity
The cost of equity is generally derived using the CAPM model that lays out the cost of equity as follows:
Ke = Rf + β (Rm-Rf)
Rf = Risk-free Rate. In economic terms, it is the rate at which an investor can earn returns without taking any risk. The US treasury bill rate can be considered a risk-free rate.
β = Beta factor. It is the sensitivity index of an asset. It indicates the magnitude of movement in the underlying asset when the market moves by 1.
Also Read: Weighted Average Cost of Capital (WACC)
Rm = Market Rate of Return. Denotes the return earned by the basket of stocks comprising the market as a whole. The Rm may also be industry-specific, like the real estate index or the utility index. It can also be a collective index such as the S&P 500.
Cost of Debt
Kd = Interest (1-t)/Value of Debt
Interest: This formula is very intuitive. It occurs naturally that the cost of debt will be the interest one pays for it. However, what is to be understood is the (1-t) factor.
Interest on borrowings is basically tax-deductible. This means that interest leads to the prevention of cash outflow in the form of taxes. Therefore, the effective cost of debt is less by the proportion of the tax component.
Cost of Preference share
The formula of Cost of Preference Share Capital:
Kp = D0/P0
D0= Dividend (generally at a fixed rate); P0= Price of preference share.
Like interest payments are a fixed obligation for debt finance, dividends are a fixed obligation for preference share capital. Since dividends are paid out of after-tax profits, they do not entail any tax shield benefits.
Also Read: WACC Calculator
WACC Calculation with Example
Lets see how to calculate WACC with an example below:
The Capital Structure of a company is as under
| Debentures ($100 per debenture) | $500,000 |
| Preference Share ($100 per share) | $500,000 |
| Equity Shares ($10 per Share) | $1,000,000 |
| $2,000,000 |
The Market prices of the securities are as under
Debentures $105 per Debenture
Preference Shares $110 per Preference Share
Equity Share $ 24 each
Additional Information
(1) $ 100 per debenture, redeemable at par, 10 % coupon rate, the applicable tax rate is 35%
(2) $100 preference share, currently trading at $ 110, 12% coupon rate.
(3) The prevailing risk-free rate on T-Bills is 5.5%. The Average market risk premium is 8%. The beta of the company is 1.8
Carrying out the WACC calculation using market value weights (You can also use book values as weights. Refer to Market vs. Book Value WACC for more).
Cost of Debentures
= Kd = Interest (1-t)/Value of Debt
= 10 (1-35%)/100 = 6.5%
Cost of Preference Shares
= Kp = Dividend Per Share/ Market Price Per Share
= $12/$110 = 10.9%
Cost of Equity Shares
= Ke = Rf + β (Rm -Rf)
= 0.055 + 1.8 (0.08-0.055)
=10 %
WACC of Capital Structure Using Market Value Weights
| Source of Funds | Market Value of Capital (A) | Weight (A/B) =C | After Tax Cost of Capital (D) | WACC (C*D) =E |
| 10 % Debentures | $525,000 | 0.151 | 6.50% | 0.98% |
| 12% Preference Share Capital | $550,000 | 0.158 | 10.90% | 1.72% |
| Equity Share Capital | $2,400,000 | 0.691 | 10% | 6.91% |
| (B) | $3,475,000 | 9.61% |
From the above WACC calculations, the net or effective cost that the business bears on its capital structure as a whole is 9.61%
Working Notes
Computation of Market Values
10% Debentures = ($500,000/100) * $105
= $525,000
12% Preference Shares = ($500,000/100) * $110
=$550,000
Equity Shares = ($1,000,000/10) * $24
=$2,400,000
For calculation, you can use our WACC Calculator.
Quiz on WACC.
RELATED POSTS
- Advantages and Disadvantages of Weighted Average Cost of Capital (WACC)
- Market vs. Book Value WACC
- Importance and Use of Weighted Average Cost of Capital (WACC)
- Project or Divisional Weighted Average Cost of Capital (WACC)
- Cost of Capital
- Evaluating New Projects with Weighted Average Cost of Capital (WACC)

