Modified internal rate of return is a solution to the shortcomings of internal rate of return as a project evaluation technique. There are two major disadvantages of IRR. One is Multiple IRR, and the other is the impractical assumption of reinvesting positive cash flows at the rate of project IRR.
Definition of Modified Internal Rate of Return
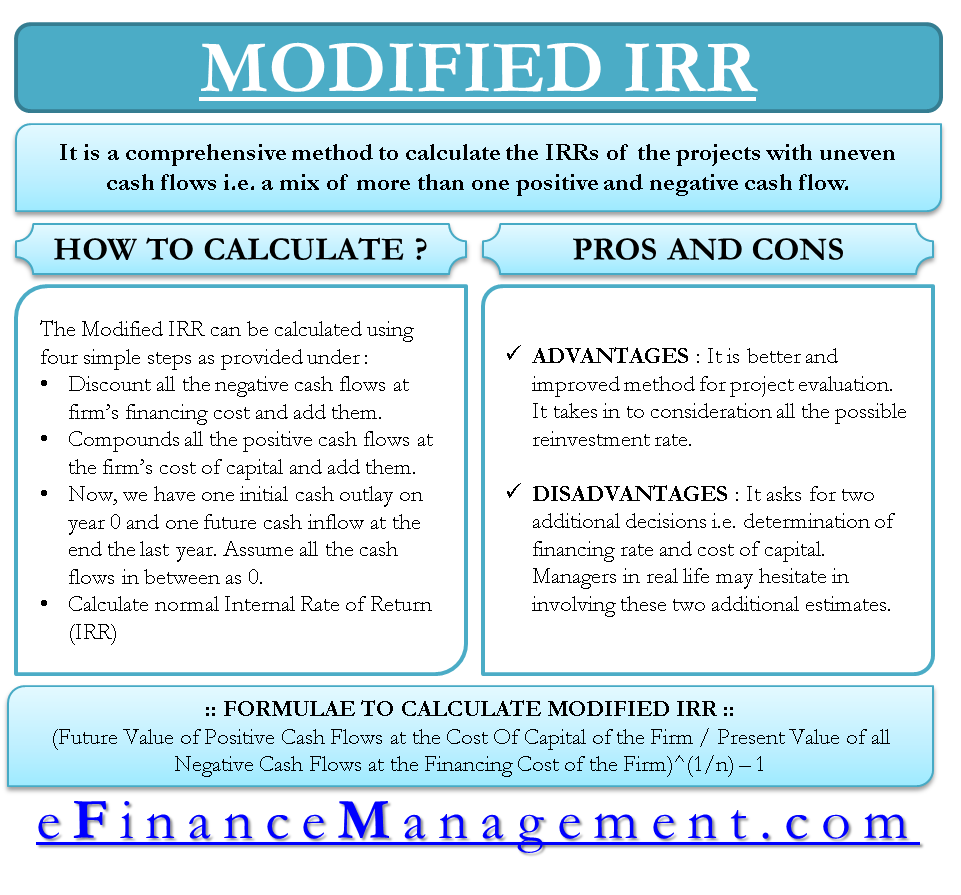
It is a comprehensive method to calculate the IRRs of projects with uneven cash flows, i.e., a mix of more than one positive and negative cash flow. It provides a solution to the above situation and assumes a practically valid reinvestment rate for positive cash flows.
Formula for Modified Internal Rate of Return (MIRR)
The formula for MIRR is as follows:
MIRR = (Future Value of Positive Cash Flows at the Cost Of Capital of the Firm / Present Value of all Negative Cash Flows at the Financing Cost of the Firm)^(1/n) – 1

How to calculate Modified Internal Rate of Return?
It looks very calculative and challenging when we see the formula for its calculation. We will make it a four-step simple solution.
- Discount all the negative cash flows at the firm’s financing cost and add them.
- Compounds all the positive cash flows at the firm’s cost of capital and adds them.
- Now, we have one initial cash outlay on year 0 and one future cash inflow at the end of the last year. Assume all the cash flows in between as 0.
- Calculate the normal Internal Rate of Return (IRR)
We will get the same result with the above steps. We will check and understand it with an example.
Modified Internal Rate of Return Explanation with Example
Consider a project with a total life of 6 years, and the fashion of cash flow is stated in the second column of the below table. The financing cost and the cost of capital are assumed at 15% and 12%, respectively. Step 1 is calculated in the third column and step 2 in the fourth column. In the last column, steps 3 and 4 are performed. The cash flow stream is rearranged with PV of negative cash flow in the 0th year and FV of positive CFs in the 6th year, and the rest all the years made 0. Using a normal method of IRR calculation, the final calculation of 19.46% is arrived at.
Also Read: Internal Rate of Return (IRR)
| Year | Cash Flows (CF) | PV of Negative CFs | FV of Positive CFs | IRR |
| 0 | -2,000.00 | -2,000.00 | -4,630.06 | |
| 1 | 1,000.00 | 1,762.34 | 0 | |
| 2 | 1,000.00 | 1,573.52 | 0 | |
| 3 | -4,000.00 | -2,630.06 | 0 | |
| 4 | 3,000.00 | 3,763.20 | 0 | |
| 5 | 3,000.00 | 3,360.00 | 0 | |
| 6 | 3,000.00 | 3,000.00 | 13,459.06 | |
| -4,630.06 | 13,459.06 | 19.46% |
Using the formula also, we get following
MIRR = (13459.06/4630.06)^(1/6) – 1
MIRR = 19.46%
Advantages and Disadvantages of MIRR
Advantage: MIRR is a better and improved method for project evaluation as it prevents all the shortcomings of normal IRR and NPV methods. It takes into consideration the practically possible reinvestment rate. The calculation is also not rocket science.
Disadvantage: The disadvantage of MIRR is that it asks for two additional decisions, i.e., determination of financing rate and cost of capital. These can be estimates again, and the managers in real life may hesitate in involving these two additional estimates.
Continue reading – Internal Rate of Return and Time-Weighted Return


For FV of CF on Y1 it should be = 1000*(1.12)^6-1 = 1972.82
FV = CF*(1+rate)^n-1
Dear Nate,
First of all thanks for taking interest in our content.
Even if you try your formula calculation again, you will get 1762.34 and not 1972.82. If you do not deduct 1 from 6, you will get 1973.82. Please recheck.
I appreciate what I got in this platform