Crossover Rate – Meaning, Formula, Example, and More

Crossover Rate is a crucial tool in capital budgeting. It primarily allows management to analyze the two mutually exclusive projects together and then decide on the better one.  

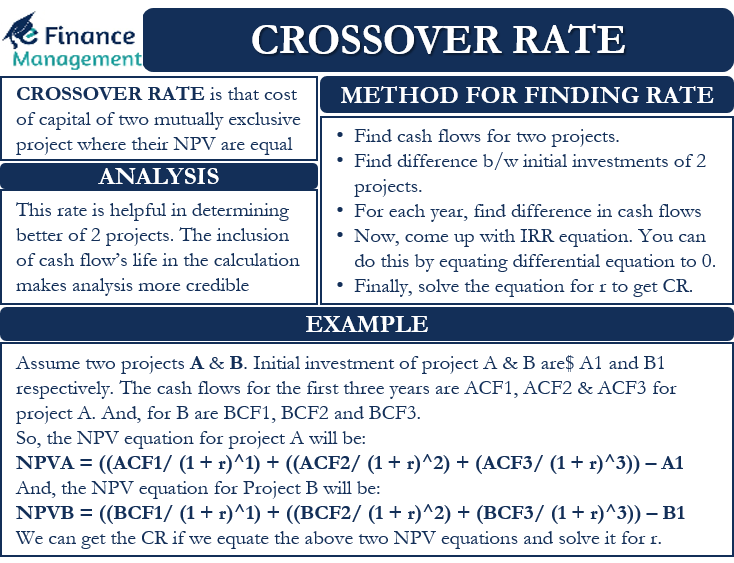
The crossover rate is the cost of capital (CoC) of two mutually exclusive projects at which their NPVs (net present values) are equal. Or it is the rate at which the NPV profiles of the two projects intersect. It means that management would be indifferent between the two projects at the crossover rate while deciding because the NPV of both the projects is exactly the same.

 This rate basically helps management to decide between two equally good projects. Or it shows why one project is better than the other project. To decide the preference, the Cost of capital becomes the yardstick. Therefore, whichever project has a crossover rate (CR) greater than its cost of capital becomes the preferred one. 

Crossover Rate Formula

The NPV is a very important input for the calculation of the CR. One can get the NPV from the present value (PV) of the project’s costs and revenues. Along with NPV, the internal rate of return (IRR) is also crucial for calculating CR. The IRR is basically the discount rate at which the NPV of expected cash flows equals zero. Usually, the more the IRR, the higher the growth potential a project has.

To understand the formula for CR, let us assume there are two projects, A and B. Project A has an initial investment of A1, while its cash flows for the first three years are ACF1, ACF2, and ACF3.

So, the NPV equation for project A will be:

NPVA = ((ACF1/ (1 + r)^1) + ((ACF2/ (1 + r)^2) + (ACF3/ (1 + r)^3)) – A1

Now assume Project B has an initial investment of B1, while its cash flows for the first three years are BCF1, BCF2 and BCF3. So, the NPV equation for Project B will be:

NPVB = ((BCF1/ (1 + r)^1) + ((BCF2/ (1 + r)^2) + (BCF3/ (1 + r)^3)) – B1

We know that CR is the rate at which the NPV of the 2 projects will be the same. So, we can get the CR if we equate the above two NPV equations and solve it for r.

Another Method

There is one more (and a shorter approach) method to get the CR. This method has the following steps:

• Find the cash flows for the two projects.
• Find the difference between the initial investments of the two projects.
• Now, for each year, find the difference in the cash flows.
• Now, come up with an IRR equation. We can do this by equating the differential equation (of cash flows and initial investments of two projects) to zero.
• Finally, we need to solve the equation for r to get the CR.

Example of Crossover Rate

A simple example will help us understand the CR calculation better under the second method. 

Assume Company A is considering two projects, X and Y. Project X would cost $50 million, while its cash flows for the first three years would be $60 million, $40 million, and $20 million, respectively. Project Y would cost $80 million and would give a cash flow of $250 million in the third year.

Now, we need to subtract the costs and cash flows of Project Y from Project X.

Initial investment Difference= $80 million less $50 million = $30 million

Difference between year 1 cash flow = $0 less $60 million = ($60 million)

Difference between year 2 cash flow = $0 less $40 million = ($40 million)

Difference between year 3 cash flow = $250 million less $20 million = $230 million.

The IRR equation now would be:

((-$60 million/(1 + r)^1) + (-$40 million/(1 + r)^2) + ($230 million//(1 + r)^3)) – $30 million = 0

Now, we need to solve the above equation to get the value of r. By solving the above equation, we will get r as 33.02%. This means Project Y is preferable if the cost of capital is less than CR (33.02%). But, if the cost of capital rises over CR (33.02%), then Project A will give a higher NPV.

Crossover Rate Analysis

As said above, the crossover rate helps determine the better of the two projects for a company. Along with the crossover rate, the inputs that go into the calculation of CR also tell a great deal about the two projects. Thus, the inputs (NPV and IRR) themselves could help evaluate the projects.

Both NPV and IRR are very popular financial metrics for evaluating projects. We use a very similar formula for calculating IRR as for NPV. However, to calculate the CR, we substitute the NPV with 0, and thus, IRR assumes the role of the discount rate. Moreover, the IRR assumes that all the cash flows get reinvested into the IRR and not the cost of capital.

Time value of money is a very important and critical concept in the financial world. Hence, we get the benefit by using the IRR as it considers this time value of money. To arrive at the present value, one needs to do the discounting for all the future cash flows (of all years). So, including the cash flow’s economic life in the calculation makes the CR analysis more credible.

However, there is one limitation of using the IRR as well. And, it is that the IRR does not consider the payback and the relative size of the investment. Thus, the calculation may not always be accurate because the CR primarily focuses on the differential. The size of the project, time span, and how fast the cash flow is coming back do have their own influence in decision making. Faster generation of cash flow is always preferred, though the total cash flow and IRR may remain the same for any two projects. 

Final Words

Now we have seen the importance of the Crossover Rate in capital budgeting decisions. It primarily helps management decide on a better project when facing a choice between two equally good but mutually exclusive projects. Moreover, the inputs (IRR and NPV) that go into the calculation of the CR are themselves important capital budgeting metrics. Thus, they add more credibility to the CR analysis.

Frequently Asked Questions (FAQs)