Modigliani- Miller Theory on Dividend Policy

Modigliani-Miller’s theory is a major proponent of the ‘dividend irrelevance’ notion. According to this concept, investors do not pay any importance to the dividend history of a company, and thus, dividends are irrelevant in calculating the valuation of a company. MM theory on dividend policy is in direct contrast to the ‘dividend relevance’ theory which deems dividends to be important in the valuation of a company.

Crux of Modigliani-Miller Model

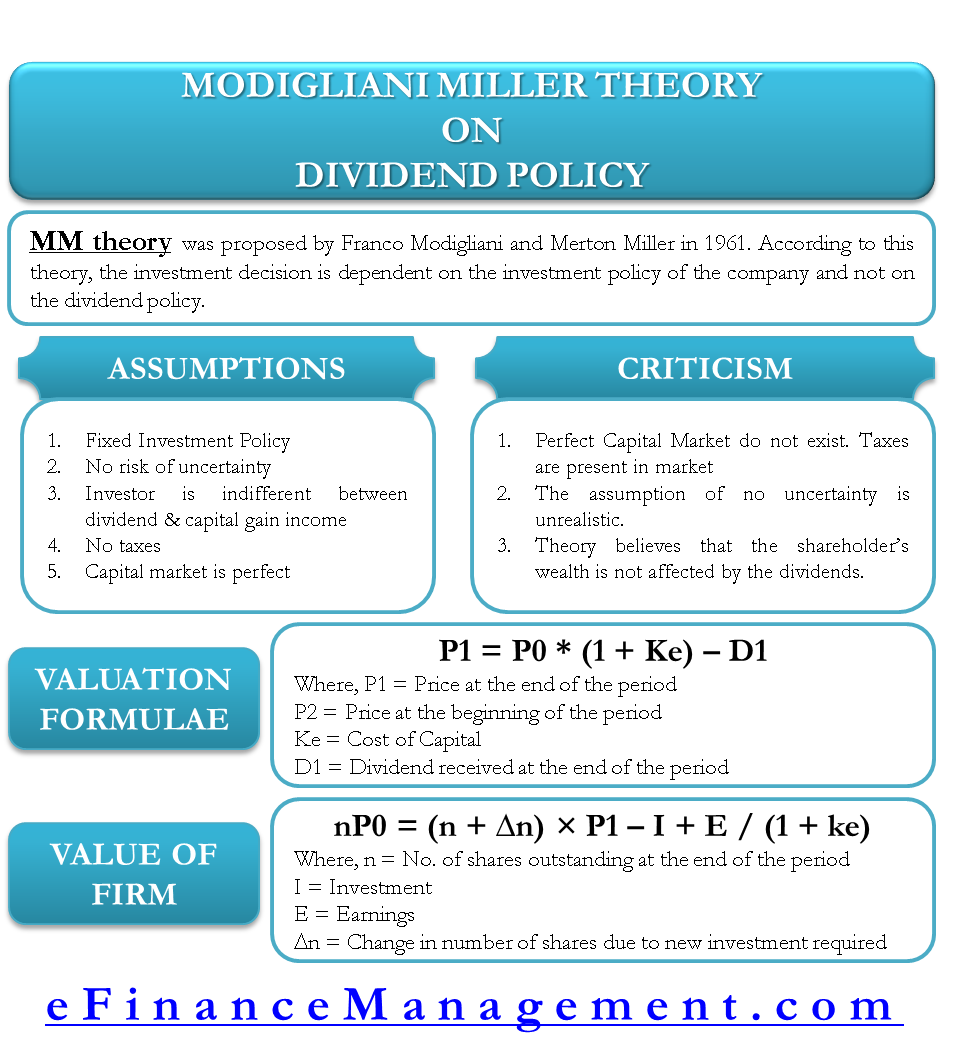
Modigliani-Miller theory was proposed by Franco Modigliani and Merton Miller in 1961. They were the pioneers in suggesting that dividends and capital gains are equivalent when an investor considers returns on investment. The only thing that impacts the valuation of a company is its earnings, which are a direct result of the company’s investment policy and future prospects. So, according to this theory, once the investor knows the investment policy, he will not need any additional input on the company’s dividend history. The investment decision is, thus, dependent on the investment policy of the company and not on the dividend policy.

MM theory goes a step further and illustrates the practical situations where dividends are not relevant to investors. Irrespective of whether a company pays a dividend or not, the investors are capable enough to make their own cash flows from the stocks depending on their need for the cash. If the investor needs more money than the dividend he received, he can always sell a part of his investments to make up for the difference. Likewise, if an investor has no present cash requirement, he can always reinvest the received dividend in the stock. Thus, the MM theory on dividend policy firmly states that a company’s dividend policy does not influence the investment decisions of the investors.

This theory also believes that dividends are irrelevant by the arbitrage argument. By this logic, external financing offsets the dividend’s distribution to shareholders. Due to the distribution of dividends, the stock price decreases and will nullify the gain made by the investors because of the dividends.

Assumptions of the Model

Modigliani-Miller’s theory is based on the following assumptions:

Perfect Capital Markets

This theory believes in the existence of “perfect capital markets.” It assumes that all the investors are rational, they have access to free information, there are no flotation or transaction costs, and no large investor to influence the market price of the share.

No Taxes

There is no existence of taxes. Alternatively, the tax rate for both dividends and capital gains is the same.

Fixed Investment Policy

The company does not change its existing investment policy. It means whatever may be the dividend payment, the company will invest as it has already decided upon. If the company is going to pay more amount of dividends, then it will have more equity shares and vice versa.

No-Risk of Uncertainty

All the investors are certain about the future market prices and the dividends. This means that the same discount rate is applicable for all types of stocks in all time periods.

Investor is Indifferent between Dividend Income and Capital Gain Income

It is assumed that investor is indifferent between dividend income and capital gain income. It means if he requires the total return of Rs. 500, he may get Rs. 200 dividend income and Rs. 300 as capital gain income or reverse. In either of the case, he gets equal satisfaction.

Valuation Formula and its Denotations

MM theory on dividend policy is based on the assumption of the same discount rate/rate of return applicable to all the stocks.

P₁ = P₀ * (1 + ke) – D1

Where,

P₁ = market price of the share at the end of a period

P₀ = market price of the share at the beginning of a period

ke = cost of capital

D1 = dividends received at the end of a period

Explanation of Modigliani-Miller’s Model

Modigliani-Miller’s model can be used to calculate the market price of the share at the end of a period if the share price at the beginning of the period, dividends, and the cost of capital are known.

The share price at the beginning of the year is Rs. 150. The discount rate applicable to the company is 10%. The company declares Rs. 10 as dividends at the end of a year. The market price of the share at the end of one year using Modigliani – Miller’s model can be found as under.

Here, P₀ = 150

ke = 10%

D1 = 10

Market price of the stock = P₁ = 150 * (1 + .10) – 10 = 150 *1.1 – 10 = 155.

Value of Entire Firm

As per MM approach, the formula for finding the value of the entire firm/company is as under:-

nP0 = (n + ∆ n) × P_{1 – I + E / (1 + ke)}

Explanation of formula

1. Retained Earning = E – n D₁

E = Earning

n = Number of Outstanding Equity shares at the beginning of the year

D₁= Dividend Paid to existing shareholders at the end of the year

2. New Issue of Equity Share Capital (Rs.) = I – Retained earning

= I – {E – n D₁}

= I – E + nD₁

I = Investment to be made at the end of the year

3. New Issue of Equity shares at the end of the year (∆n)

New Issue of Equity Shares at the end of year = ∆n × P₁

So, we can say that

∆n × P_{1 = New Issue of Equity Share Capital (Rs.)}

∆n × P_{1 = I – E + nD1}

Now, explanation of the formula starts,

As we know that for one equity share:-

P₁ = P₀ * (1 + ke) – D1

P₀ = D₁ + P1 / (1 + ke)

Now, in the above equation, multiply both sides by n, so instead of one share, it will become the value of the firm:-

nP₀ = nD₁ + nP1 / (1 + ke)

In order to derive a formula, ∆n × P₁ is added and subtracted to right hand side equation:-

nP₀ = nD₁ + nP1 + ∆n × P_{1 –  ∆n × P1}/ (1 + ke)

Now, P1 is taken common from nP1 and ∆n  P₁

nP₀ = nD₁ + (n + ∆n) × P_{1 –  ∆n × P1}/ (1 + ke)

Replacing ∆n × P₁ with  I – E + nD₁

nP₀ = nD₁ + (n + ∆n) × P_{1 –  {I – E + nD1}}/ (1 + ke)

Now, we open bracket of I – E + nD₁

nP₀ = nD₁ + (n + ∆n) × P_{1 –  I + E – nD1}/ (1 + ke)

Cancelling nD1 from both sides; we are left with following formula :-

nP₀ = + (n + ∆n) × P_{1 –  I + E} / (1 + ke)

Criticism of Modigliani Miller’s Model

MM theory on dividend policy suffers from the following limitations:

• Perfect capital markets do not exist. Taxes are present in the capital markets.
• According to this theory, there is no difference between internal and external financing. However, on considering the flotation costs of new issues, it is false.
• This theory believes that the dividends do not affect the shareholder’s wealth. However, there are transaction costs associated with the selling of shares to make cash inflows. This makes the investors prefer dividends.
• The assumption of no uncertainty is unrealistic. The dividends are relevant under certain conditions as well.

Conclusion

Modigliani – Miller’s theory of dividend policy is an interesting and different approach to the valuation of shares. It is a popular model that believes in the irrelevance of dividends. However, the policy suffers from various important limitations and thus, is critiqued regarding its assumptions. The bird in hand theory by Myron Gordon and John Lintner is in response to this theory and talks about investors’ concern in preferring dividends rather than capital gains.