What are Laspeyeres and Paasche Indexes?
Laspeyres and Paasche Indexes are the two most popular indexes to calculate the changes in the price level over time. Both these indexes use the prices of commodities in the observation year and the base year to come up with the changes in the price level between these periods.
Importance of Laspeyers and Paasche Indexes
To ascertain, decipher and take suitable actions based on price level changes of various commodities and baskets of commodities over a given period is an important and critical part of the economics and fiscal policy formulation.
Difference between Laspeyres vs Paasche Index
Though these two measures appear similar, there is a slight difference in their methodology of calculation. This slight difference leads to many more differences between the Laspeyres vs Paasche Index. These differences are with regard to their results and interpretations. In this article, let us attempt to understand and appreciate the differences between the Laspeyres Vs Paasche Index.
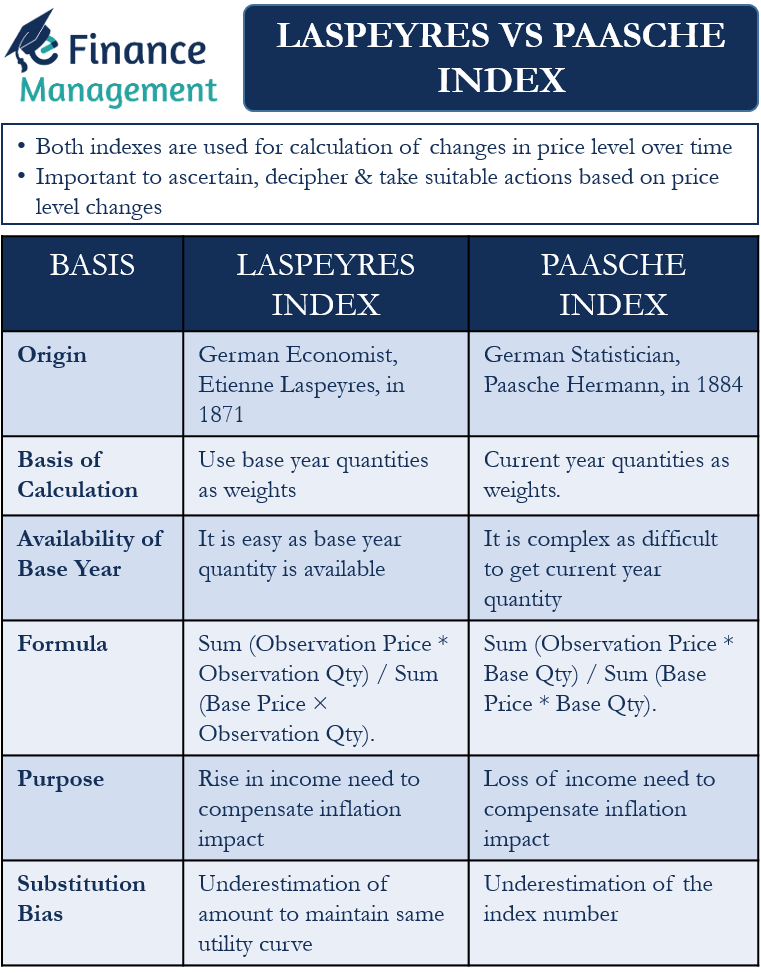
Origin
German Economist, Etienne Laspeyres, was the first to come up with the Laspeyres Index method 1871. And German Statistician, Paasche Hermann, was the first to come up with the Paasche Index method 1874.
Basis of Calculation
As said above, both the indexes use the prices of commodities in the observation year and the base year. However, the weights or the quantity of commodities they consider make them very different from each other.
Laspeyres Index uses the base year quantities as weights. And the Paasche Index uses the current year quantities as weights.
So, we can say Laspeyres Index calculates how much the same amount of commodities or services in the base year would cost in the observation year. And the Paasche Index considers how much the observation period commodities would have cost in the base year. In simple words, the Laspeyres Index calculation approach is from bottom to top approach (base year to observation Year). At the same time, Paasche Index does exactly reverse – observation year to base year.
Availability of Base Year Quantities
Even though both the indexes use price and quantity data, the Laspeyres index is easier than the other one. This is because the base-year quantities are easily available. On the other hand, for the Paasche index, we need to search for the current year quantities or how the consumption has changed over time. Moreover, due to substitution or new product or technology launch, it would become difficult to compare the same in the base year as those products and services may not be present. Secondly, the prices will also not give a true representation because any new product pricing will differ in the initial period of launch vs. when it settles down. It could be either very cheap or high at the time of launch.
Formula
The formula to calculate the Paasche Index is Sum (Observation Price * Observation Qty) / Sum (Base Price × Observation Qty).
The formula to calculate the Laspeyres Index is Sum (Observation Price * Base Qty) / Sum (Base Price * Base Qty).
Calculation
The way to calculate both these indexes is to get the ratio of the sum of prices (of base and current year) multiplied by the quantities. So, for the Laspeyres index, the numerator is the sum of the current prices multiplied by the base year quantities. And the denominator is the base year prices multiplied by the base year quantities.
Similarly, for the Paasche index, the numerator is the sum of the current prices multiplied by the current year quantities. But the denominator consists of current year quantities multiplied by base year prices.
Purpose
The Laspeyres index uses the base year quantities. Hence, it represents how much one’s income needs to rise to compensate for the rise in the prices for the same basket of commodities over the period.
And the Paasche index uses current quantities. Therefore, it indicates how much income one needs to lose at the base price level to compensate for the impact of inflation on the consumption of the same basket of commodities.
Substitution Bias
This is the biggest drawback of both indexes. Both these indexes ignore the impact on the quantities of the products or basket of products due to the substitution product availability during the period. Thus, both of these indexes do not recognize the change in the consumption pattern on account of substitution possibilities. It is basically the consumer behavior that suggests consumers go for cheaper substitutes when the cost of an item goes up.
Since the Paasche index considers the current quantities, overlooking the substitution effect results in the underestimation of the index number.
And, since the Laspeyres index considers the base year quantities, it tends to underestimate the amount that one would need to maintain the same utility curve in the observation year as the base year.
Owing to this substitution effect, many economists use the Fischer index to calculate inflation. The Fischer index is the square root of the product of the Paasche and Laspeyres index. In this way, the Fischer index eliminates the substitution bias.
Final Words
Both Laspeyres and Paasche Index are popular measures of the changes in the price level or inflation of the index constituent commodities. Many economists, however, do not use it because they do not consider the substitution effect. To overcome this drawback of the Laspeyres and Paasche Index, many economists use the Fischer index, which is the square root of these two indexes.

