Why Must Marginal Utility be Equal to Price?

Marginal Utility is the extra or additional utility or satisfaction that a user gets after consuming one more unit of a commodity. The concept of MU is very useful in understanding consumers’ buying decisions, given their limited income. Also, in economics books, you would have read that it is important for consumers to keep consuming until the marginal utility equals the price of the goods or services. But why must marginal utility be equal to price?

In a nutshell, we can say that to reach optimal consumption; the marginal utility must be equal to the price. But exactly how equating marginal utility and price gives optimal consumption is something we will be answering in this article.

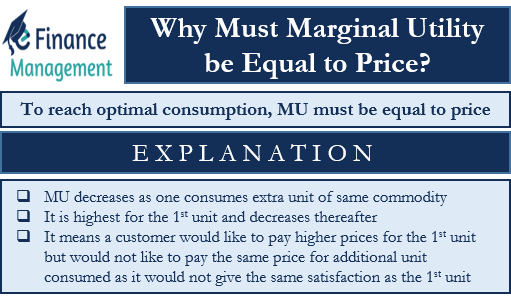
Explanation: Why Must Marginal Utility Equal to Price?

Marginal utility (MU) isn’t constant; in fact, it keeps on diminishing as we consume more units of the same commodity. The MU is generally highest for the first unit and drops as a user consumes more units of the same commodity. We call this the law of diminishing marginal utility.

Depending on the number of units that one consumes, the marginal utility can be positive, zero, or even negative.

Suppose you are thirsty and you like an energy drink, then the first unit will give you maximum satisfaction. Now, if you still want to drink another unit, then the MU of the energy drink will be positive. MU will be zero if consuming another energy drink won’t bring any satisfaction or joy to you. And MU will be negative if you don’t feel like drinking any more energy drinks or consuming more will get you sick.

One can easily relate this diminishing MU concept with the concept of diminishing prices as well. If you continue to consume more of a commodity, its MU will drop. Along with the drop in MU, the price that you could be willing to pay for that extra unit will drop as well.

Taking the above energy drink example, you could be willing to pay a higher price for the first unit of energy drink because you are thirsty. After the first unit, you don’t feel that thirsty, so you may not be willing to pay the same price that you could have paid for the first unit. So, we can say that the optimum level of consumption is to consume a product or service until the point where the MU equals the price.

Suppose we measure utility in terms of dollars spent, i.e., the money that a user will be willing to spend to buy an additional unit. Now it would be illogical to pay the price for that commodity if the satisfaction you get is less than that.

Example

Taking the energy drink example above, suppose the price of one energy drink is $10. If you are very thirsty, then you could be willing to pay extra to get the energy drink, say $20. The MU for the first energy drink ($20) is more than its price.

Now, you are not that thirsty, but you still feel the urge to drink another energy drink. You are ready to pay $15 for it. For the second energy drink also, the MU ($15) is more than the price.

Now, you are not thirsty, but you can still drink another energy drink. But now you are ready to pay $10 for it. In this case, the MU of the third energy drink is equal to its price. So, this will be optimum consumption.

In real life also, there are a lot of examples that use such a concept. Ticket pricing by Airlines does reflect the marginal utility concept. The prices go up as the journey date approaches. Since the marginal utility for a passenger will be more due to any medical emergency, unplanned trip, etc., they would be willing to pay a higher price if they book the ticket close to the journey date.